그래프이론 |
그래프와 트리에 관련된 알고리즘인 만큼 그래프와 트리 자료구조에 대한 이해가 먼저 필요함
그래프 : https://smallpants.tistory.com/190
트리 : https://smallpants.tistory.com/189
그래프 순회(탐색)알고리즘은 여러종류가 있는데 그 중 대표적인 것이 BFS와 DFS이다.
또한 그래프에는 많은 종류가 있으며 그 중 하나인 **무방향 연결그래프**가 트리이다.
즉 트리는 그래프의 일종이며, 그러므로 BFS나 DFS같은 탐색은 그래프에도, 트리에도 적용할 수 있다.
BFS와 DFS의 용도 및 장단점 |
BFS
- 용도 : 모든 간선의 가중치가 같은 그래프에서 최단거리 탐색
- 장점
1) 너비를 우선으로 탐색하므로 답이 되는 경로가 여러가지여도 최단 경로임이 보장됨
2) 최단 경로가 존재한다면, 어느 한 경로가 무한히 깊어진다 해도 반드시 최단 경로를 찾을 수 있음
3) 탐색하고자 하는 노드의 위치가 얕을 때 유리함
4) 노드의 갯수가 적을때 유리함
- 단점
1) "노드의 갯수가 많은 경우" 다음에 탐색할 노드들을 모두 Queue에 저장하기 때문에 필요없는 노드들까지 저장해야함
즉 저장 공간이 많이 필요해짐
DFS
- 용도 : 완전탐색, 백트래킹
- 장점
1) BFS에 비해 저장공간의 필요성이 적음, 백트래킹을 해야하는 노드들만 저장해주면 됨
2) 탐색하고자 하는 노드의 위치가 깊을수록, 그 노드가 좌측에 있을수록 BFS보다 유리함
- 단점
1) 찾고자 하는 노드가 없는 경로를 탐색할 때 그 경로가 매우 깊다면 쓸데없는 비효율이 발생함
2) 찾은 경로가 최단경로라는 보장이 없음
BFS |
1. 개념
너비우선탐색(BFS, Breadth First Search)
이름처럼 너비를 우선순위로 탐색하는 방식
먼저 같은 레벨에 있는 노드를 모두 탐색한 후 다음 레벨의 노드로 이동해서 탐색하는 방식
트리순회 방법 중 레벨순회에 해당
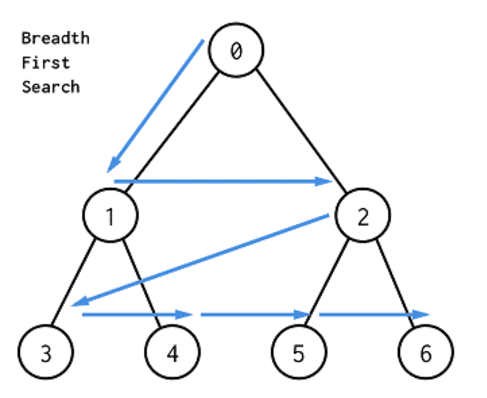
위 트리를 BFS로 탐색한다면 탐색순서는 0 -> 1 -> 2 -> 3 -> 4 -> 5 -> 6이 되는 것
2. 구현
BFS는 Queue를 이용해서 구현
쉽게 생각하려면 방문된 노드는 큐에 들어감
그 다음 큐에서 값을 빼서 해당 값의 자식노드들이 방문되었었는지 검사받음
그리고 그 자식노드들 또한 방문됐으니 큐에 들어감
#include <iostream>
#include <vector>
#include <queue>
std::vector<bool> visited;
std::vector<std::vector<int>> adj;
void bfs(int u)
{
visited[u] = true;
std::cout << u << ' ';
std::queue<int> q;
q.push(u);
while (q.size()) {
int here = q.front();
q.pop();
for (int there : adj[here]) {
if (visited[there]) continue;
visited[there] = true;
std::cout << there << ' ';
q.push(there);
}
}
}
int main()
{
std::ios::sync_with_stdio(false);
std::cin.tie(NULL);
int number_of_vertex, number_of_edge, start_vertex;
std::cin >> number_of_vertex >> number_of_edge >> start_vertex;
visited.resize(number_of_vertex + 1);
adj.resize(number_of_vertex + 1);
int u, v;
for (int i = 0; i < number_of_edge; i++) {
std::cin >> u >> v;
adj[u].push_back(v);
adj[v].push_back(u);
}
bfs(start_vertex);
return 0;
}
DFS |
1. 개념
깊이우선탐색(DFS, Depth First Search)
이름처럼 깊이를 우선순위로 두고 탐색하는 방식
한 방향으로 끝까지 내려가서 탐색을 하고 더이상 탐색할 곳이 없으면 한칸 위로 올라와서 다른 깊이로 탐색하는 방식
트리순회 방법 중 전위순회에 해당
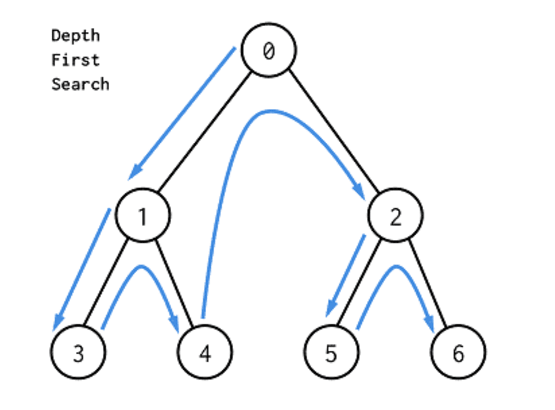
위 트리를 DFS로 탐색한다면 탐색 순서는 0 -> 1 -> 3 -> 4 -> 2 -> 5 -> 6이 되는 것
2. 구현
DFS는 재귀함수를 이용해서 구현하고 두 가지 방법으로 나뉘며 둘 다 사용할 줄 알아야 함
1) 다음 vertex가 방문됐었는지 검사 한 후 가는 방식
2) 무작정 가보고 방문됐었던 곳이면 그냥 return하는 방식
1번방식(노크하고 진입)
void dfs(int here){
visited[here] = 1;
for(int there : adj[here]){
if(!visited[there]) dfs(there);
}
}
2번방식(무작정 진입)
void dfs(int here){
if(visited[here]) return;
visited[here] = 1;
for(int there : adj[here]){
dfs(there);
}
}
1번방식으로 구현해본 전체 코드
#include <iostream>
#include <vector>
std::vector<bool> visited;
std::vector<std::vector<int>> adj;
void dfs(int u)
{
visited[u] = true;
std::cout << u << ' ';
for (int v : adj[u]) {
if (!visited[v]) dfs(v);
}
}
int main()
{
std::ios::sync_with_stdio(false);
std::cin.tie(NULL);
int number_of_vertex, number_of_edge, start_vertex;
std::cin >> number_of_vertex >> number_of_edge >> start_vertex;
visited.resize(number_of_vertex + 1);
adj.resize(number_of_vertex + 1);
int u, v;
for (int i = 0; i < number_of_edge; i++) {
std::cin >> u >> v;
adj[u].push_back(v);
adj[v].push_back(u);
}
dfs(start_vertex);
return 0;
}
3. 연결된 컴포넌트 탐색
몇개의 구역으로 나뉘어져있는지 탐색하는 문제다.
예시 문제)
기초버전 : https://smallpants.tistory.com/265
심화버전 : https://smallpants.tistory.com/263
예시 코드)
#include<bits/stdc++.h>
using namespace std;
int dy[4] = {-1, 0, 1, 0};
int dx[4] = {0, 1, 0, -1};
int m, n, k, y, x, ret, ny, nx, t;
int a[104][104];
bool visited[104][104];
void dfs(int y, int x){
visited[y][x] = 1;
for(int i = 0; i < 4; i++){
ny = y + dy[i];
nx = x + dx[i];
if(ny < 0 || nx < 0 || ny >=n || nx >= m) continue;
if(a[ny][nx] == 1 && !visited[ny][nx]){
dfs(ny, nx);
}
}
return;
}
int main(){
cin >> n >> m;
for(int i = 0; i < n; i++){
for(int j = 0; j < m; j++){
cin >> a[i][j];
}
}
for(int i = 0; i < n; i++){
for(int j = 0; j < m; j++){
if(a[i][j] == 1 && !visited[i][j]){
ret++; dfs(i, j);
}
}
}
cout << ret << '\n';
return 0;
}
트리순회 |
트리순회(Tree traversal)는 트리 구조에서 각 노드를 정확히 한번만, 체계적으로 방문하는 과정을 의미
방문순서에 따라 전위순회, 중위순회, 후위순회, 레벨순회가 있음
설명은 이진트리 기반으로 되어있지만 다른종류의 트리에서도 일반화시킬 수 있음
1) 전위순회(preorder traversal)
전위순회는 먼저 자신의 노드를 방문하고 그 다음 노드들을 방문하는 방식
일반적인 DFS의 모습
수도코드
preorder( node )
if (node.visited == false)
node.visited = true
preorder( node->left )
preorder( node->right )
2) 중위순회(inorder traversal)
왼쪽 노드를 먼저 방문 후 자신의 노드를 방문하고 그 다음 오른쪽 노드를 방문하는 방식
수도코드
inorder( node )
if (node.visited == false)
inorder( node->left )
node.visited = true
inorder( node->right )
3) 후위순회(postorder traversal)
자식들 노드를 먼저 방문한 후 자신의 노드를 방문하는 방식
수도코드
postorder( node )
if (node.visited == false)
postorder( node->left )
postorder( node->right )
node.visited = true
4) 레벨순회(level traversal)
일반적인 BFS의 모습
루트로부터 위에서 아래로 레벨순으로 순회하므로
5) C++ 기반의 전위순회/중위순회/후위순회
#include <bits/stdc++.h>
using namespace std;
vector<int> adj[1004];
int visited[1004];
void preOrder(int here) {
if(visited[here] == 0) {
visited[here] = 1;
cout << here << ' ';
if(adj[here].size() == 1)
preOrder(adj[here][0]);
if(adj[here].size() == 2) {
preOrder(adj[here][0]);
preOrder(adj[here][1]);
}
}
}
void inOrder(int here) {
if(visited[here] == 0) {
if(adj[here].size() == 1) {
inOrder(adj[here][0]);
visited[here] = 1;
cout << here << ' ';
}
else if(adj[here].size() == 2) {
inOrder(adj[here][0]);
visited[here] = 1;
cout << here << ' ';
inOrder(adj[here][1]);
}
else {
visited[here] = 1;
cout << here << ' ';
}
}
}
void postOrder(int here) {
if(visited[here] == 0) {
if(adj[here].size() == 1)
postOrder(adj[here][0]);
if(adj[here].size() == 2) {
postOrder(adj[here][0]);
postOrder(adj[here][1]);
}
visited[here] = 1;
cout << here << ' ';
}
}
int main(){
adj[1].push_back(2);
adj[1].push_back(3);
adj[2].push_back(4);
adj[2].push_back(5);
int root = 1;
cout << "\n 트리순회 : postOrder \n";
postOrder(root); memset(visited, 0, sizeof(visited));
cout << "\n 트리순회 : preOrder \n";
preOrder(root); memset(visited, 0, sizeof(visited));
cout << "\n 트리순회 : inOrder \n";
inOrder(root);
return 0;
}
출처